In previous posts, we have established that two sets are of the same size if there is a one-to-one correspondence between the elements of both sets. Applying this principle to Cantor’s theory of infinity leads us to the weird but valid conclusion that the number of points on a line segment is the same as the number of points in a square. To show that this is true, here is a picture of a unit length line segment and a unit square.

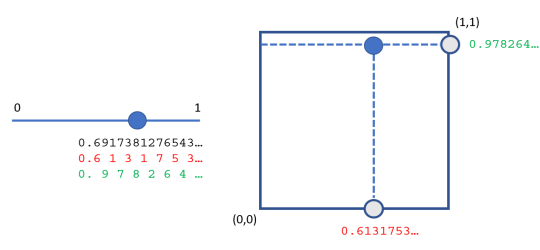
Let’s choose a point on the line segment. Let’s say 0.6917381276543… . It’s shown with a big blue dot on the line segment on the left. If this point corresponds to an irrational number, it goes on forever without repeating or showing a discernible pattern.

We’re going to break up this number into two numbers. The first number is every other digit. Shown in red below the line segment is this number, namely 0.6131753… . The remaining digits form the second number, shown in green. It is 0.978264… .

A single number between zero and one can thus be broken into two numbers between zero and one. We can take these two numbers and as the x and y coordinates on the unit square shown on the right. They define the blue dot shown in the square on the right. So the blue dot on the line segment maps to the blue dot in the square.

For every point on the line segment, there is one and only one blue dot in the square. The size of the sets of infinity on a line segment and in a square are exactly the same. An extension of this argument shows that the number of points in a cube is the same as the number of points on a line segment. This is counterintuitive and weird.

Backward mapping from the square to the line segment is also possible. Take the two coordinates defining the blue dot in the square and shuffle them together to obtain one number. Two numbers between zero and one can always be combined to make a single number between one and zero. This new number is the point on the line segment.

Since there is a one-to-one mapping of every point on the line segment to every point in the square, the number of points on a line segment is the same as the number of points in a square.

But can’t you draw a line segment and square on a sheet of paper and do the mapping? Isn’t this an illustration of infinity in reality? No. Each point is assumed to be measured with infinite precision. Precision in reality is always finite. So the conclusion that there are no infinities in reality stands strong.

This counterintuitive result, driven by Cantor’s theory of infinities is strange. Nevertheless, it is a valid property of the infinite.

Next: We’ll show that the entire Library of Congress is digitally encoded on almost every randomly chosen number.

We hope you enjoy this series on the unique, reality-defying nature of mathematical infinities. Here are all five parts — and a bonus:

Part 1: Why infinity does not exist in reality. A few examples will show the absurd results that come from assuming that infinity exists in the world around us as it does in math. In a series of five posts, I explain the difference between what infinity means — and doesn’t mean — as a concept.

Part 2. Infinity illustrates that the universe has a beginning. The logical consequences of a literally infinite past are absurd, as a simple illustration will show. The absurdities that an infinite past time would create, while not a definitive mathematical proof, are solid evidence that our universe had a beginning.

Part 3. In infinity, lines and squares have an equal number of points Robert J. Marks: We can demonstrate this fact with simple diagram. This counterintuitive result, driven by Cantor’s theory of infinities is strange. Nevertheless, it is a valid property of the infinite.

Part 4. How almost any numbers can encode the Library of Congress. Robert J. Marks: That’s a weird, counterintuitive — but quite real — consequence of the concept of infinity in math. Math: Almost every number between zero and one, randomly chosen by coin flipping, will at some point contain the binary encoding of the Library of Congress.

and

Part 5: Some infinities are bigger than others but there’s no biggest one Georg Cantor came up with an ingenious proof that infinities can differ in size even though both remain infinite. In this short five-part series, we show that infinity is a beautiful — and provable — theory in math that can’t exist in reality without ludicrous consequences.

You may also wish to read: Yes, you can manipulate infinity in math. The hyperreals are bigger (and smaller) than your average number — and better! (Jonathan Bartlett)