Many people learn to hate math early on. One of the places where people learn to hate math first is in high-stakes speed testing for math facts. This has caused quite a bit of angst in mathematics education for people on both sides of this issue. On the one hand, some have advocated for getting rid of math facts memorization altogether. On the other hand, others have doubled-down, saying that we need speed tests in order to make sure that the cognitive load of arithmetic is limited for later mathematics work. While I fall more into the latter camp than the former, I do think that a more balanced approach to mathematics education may help students in the long run. 

The goal of this article is to provide resources and ideas for parents and teachers to help students achieve mastery of the basics they will need for the future. 

The fact is, students need to know their math facts. One of the best predictors of algebra success is how well and fast students can do arithmetic. Basic algebra is just thinking a little more deeply about the way that arithmetic works and how you can work arithmetic problems backwards if the question is asked a little differently. Therefore, being at ease with arithmetic provides students with a background that helps them move into algebra.

Unfortunately, math facts are oftentimes taught merely as tables to memorize when there are, in fact, innumerable ways to teach math facts that help make it more fun, as well as help prepare students for deeper thinking later.

Learning Facts in a Deeper Way

One way to help students learn math facts is by simply using dots on a flashcard, and asking students both (a) what math problem does this represent? and (b) what is the answer? An example of this is below:

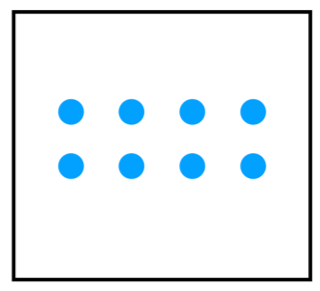
This can represent a number of different math problems: 2×4=8, 4×2=8, 4+4=8, and 2+2+2+2=8. Additionally, it represents the connection between these concepts as well. Asking students to look at the dots and think of the different equations it can represent help solidify important facts about mathematics and logic.

Additionally, this card itself has the answer on it. Students who are not yet proficient with their mathematics tables can literally count the number of dots to find the answer. This provides an activity that a student of any level can get correct, while also helping students make deeper connections within mathematics.

You can even demonstrate commutative properties of addition and multiplication by simply turning the card sideways:

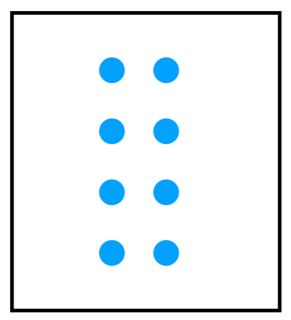
Without having to use technical jargon such as “the commutative property of multiplication,” students can intuitively see that turning the card on its side is not affecting the answer. Turning the card on its side has not changed the number of dots, and that is why 2×4 is the same as 4×2.

Additionally, getting students to look at the dots and develop a math equation (i.e., 2×4=8) helps students relate mathematics to real things. They can see the dots, and being able to relate the numbers to the dots helps connect the concrete and the abstract.

Skip-Counting

Another way to do basic memorization is skip-counting. Students usually intuitively understand skip-counting. Students know how to count, and then they can easily be taught to skip-count through the numbers they know. 

For instance, skip-counting the twos is “2, 4, 6, 8, 10, 12, 14, …” This is the same as the multiplication table for twos, but is easier to remember, because it is so close to counting.

Additionally, some people have developed songs for skip-counting, which makes it even easier to memorize. Younger students tend to be very good at memorizing, especially memorizing songs. Teaching skip-counting to music helps students bring addition and multiplication together in an easy way. They can recognize that 2×3 is just hitting the third number in the skip-counting by twos song.

Speed of Facts Through Games

Speed of recall is also important. The best way to practice recall speed is through games. A number of games have been developed which helps the speed of facts. A straightforward one is just using 10-sided dice. Roll the dice, and have the student add and/or multiply the numbers. Using dice makes it more fun and more like a game.

Another fun game for more advanced students is “number knock-out.” In this game, a series of numbers (usually 1 through 36) are written on the board. The student roles three dice, and must construct mathematical formulas using the numbers on the dice which result in one of the numbers on the board. So, if you roll a 2, 3, and 4, you can scratch off 9 by adding them all together, scratch off 14 by doing 3×4+2, and scratch off 24 by doing 2x3x4. 

More advanced students can add the operations available to them in number knock-out. Adding in powers, roots, and logarithms can increase the number of numbers that can be crossed off in a given roll. 

Number knock-out can be played competitively, cooperatively, in teams, etc. Students sometimes work forward by simply putting arbitrary operations in between the numbers, and sometimes work backward by looking at the numbers they need to form, and try to think of a way of doing this with the numbers they rolled on the dice. Number knock-out encourages both forward and reverse thinking, speed, and creativity in mathematics.

Evaluation

The one place where I agree somewhat with the “anti-math-facts” group is in evaluation. Timed tests help teachers understand where the students are at. However, high-stakes timed tests are major stressors for students. Being able to be quick with math facts makes algebra a lot easier, but I’m not sure that being able to fill out a complete multiplication table in 2 minutes vs 3 minutes is all that helpful. What is perhaps more helpful is to have a student list out the math facts that they can do instantly, and then use the blank spots as the basis for where they need more practice.

Do we need evaluation? Indeed we do, but poor test performance on math facts at one particular time in your life should not permanently put you in the “bad at math” category. The goal of evaluation is for students to know what they need help on and then receive that help. The goal of evaluation should not be in developing a permanent intellectual underclass (which high-stakes testing sometimes does), but specifically in identifying the things that someone needs to work on in order to fully succeed.

Some have claimed that timed math facts tests lead to long-term math anxiety. However, the evidence for this is lacking. Indeed, research has shown that tests in general do lead to stress, but we don’t really need research to know that.

I think that one reason for high-stakes evaluation is that the goal may be to leave “fact-learning” behind. However, I think that fact-learning is something that can be incorporated and reinforced throughout elementary mathematics education. In fact, I’ve found that “Number Knock-out” is fun even for high school students.

In short, we need tests to know where students need help, but we shouldn’t use them to close the book on math facts practice.

You may also wish to read:

Bartlett’s calculus paper reviewed in mathematics magazine. The paper offers fixes for long-standing flaws in the teaching of elementary calculus.