The NBA 3-point contest is coming up fast. Last year’s contest in Charlotte, North Carolina, featured brothers Steph and Seth Curry, who grew up in Charlotte, cheered on by their father Dell, who once played for the Charlotte Hornets. Steph made it to the championship round but Joe Harris won, making 19 of 25 shots, including an astounding 12 in a row. The buzzword on social media was hot. Harris definitely got hot. Or did he?

Most players and fans believe that players can get hot hands, effortlessly making shot after shot. Purvis Short, who once scored 59 points in an NBA game, said that, “You’re in a world all your own. It’s hard to describe. But the basket seems to be so wide. No matter what you do, you know the ball is going to go in.”

Three skeptical professors—Thomas Gilovich, Robert Vallone, and Amos Tversky—looked at 48 Philadelphia 76ers home games some years ago and concluded that “hot hands” is a myth. Streaks of makes and misses happen no more often than do streaks of heads and tails in coin flips. Several players actually did slightly worse after making a shot than after missing one (51% versus 54%).

Their myth-busting paper is justly famous. But there are good reasons for being skeptical of the skeptical professors. Unlike coin flips, basketball shots are not taken one after another from the same location. Some shots are taken several minutes apart or even in different halves of a game. Some shots are long-range bombs, others are tomahawk dunks. Players might do worse after making several shots in a row because they are lured into trying more difficult shots.

The NBA’s annual 3-point shooting contest is not only entertaining; it gives us a chance to look for hot hands under reasonably controlled conditions. The invited players take turns attempting 25 long-range shots, separated into 5 shots from each of 5 stations. The top 3 shooters from a qualifying round move on to the championship round, where they again attempt 25 shots. However, this year’s contest is going to add more long-range shots.

When Harris made 12 shots in a row during the 2019 contest, was he hot or is he just a very good shooter? Harris is a terrific 3-point shooter. He led the league during the regular season, making 47.4 percent of his 3-point shots. The All-Star competition offers more opportunity for a shooter than the regular season would because there are no defenders. Harris made 36 of 50 (72 percent) of his attempts in the qualifying and championship rounds.

The probability that anyone, even someone as good as Harris, will make 12 out of 12 shots is very low, in fact less than 2 percent—and well below the usual 5 percent threshold that statisticians consider significant.

Not so fast. Harris’ streak started with his third shot. We should calculate the probability that he would make 12 in a row at some point in his 25 shots. Given that he made 19 shots and missed 6, the chance of a streak of at least 12 is 7 percent.

Even this 7 percent calculation is misleading. There were 10 players and 13 sets of 25 shots (10 qualifying sets and 3 championship sets). It is cherry-picking to focus on Harris’ championship set after the results are in and pretend that we didn’t look at the other 12 sets. The correct question is, what is the probability that, by chance alone, at least one of the 13 sets would feature a streak that has less than a 5 percent chance of occurring. That probability works out to be a sobering 49 percent.

When we consider all the data for the 2019 contest, we see that it is as likely as not that at least one player will have a statistically impressive streak. The fact that the best streak we found was not quite statistically significant is, if anything, evidence against the claim that basketball players get hot hands.

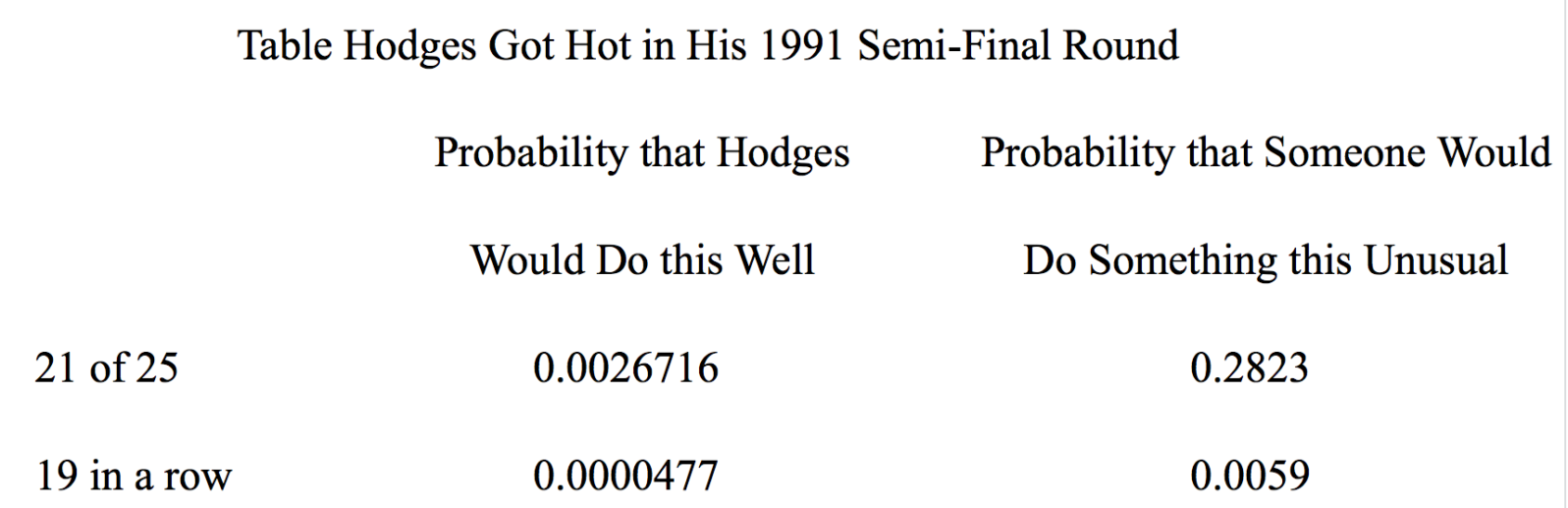
But what about other players in the history of the 3-point competition, which started in 1986? Since then, 124 players have taken a total of 389 25-shot sets. The most remarkable performance was in 1991 when Craig Hodges made 21 of 25 shots in the semi-final round, including an astonishing 19 in a row—both all-time records.

Hodges played in the NBA for 10 years and led the league in 3-point accuracy three times. He participated in the 3-point contest eight times, winning three times and finishing second twice. He was a great 3-point shooter and we have 19 rounds of contest data for him. Did Hodges get hot in the 1991 semi-finals or was his performance merely consistent with his ability?

Overall, Hodges made 268 out of 475 shots (56%). Our first question is, for a player who made 268 of 475 shots in 19 rounds, what is the probability that he would (a) have at least one round where he made 21 of 25 shots, and (b) have at least one round where he made 19 shots in a row? Our second question is, taking into account that 124 players have participated in the contest, what is the probability that at least one player would do something as remarkable as Hodges did in 1991?

The answers are below. First, given Hodges’ overall performance, there is a very low probability that he would do as well as he did in his 1991 semi-final round, particularly his 19-shot streak. Second, given that 124 players have participated in the contest, there is a low probability that any player would do something as unlikely as Hodges’ 19-shot streak:

It is certainly not straightforward to assess hot and cold streaks! Superficial evidence, like someone making several shots in a row, may well be due to chance—like heads coming up four times in a row in ten coin flips. Even when we identify something as memorable as Joe Harris making 12 shots in a row in 2019, it might be explained by the fact that we cherry-picked—like flipping ten coins 1,000 times and noting that heads once came up ten times in a row.

Fortunately, there are ways to account for chance and cherry-picking and, when we do, Craig Hodges’ streak of 19 in a row in the 1991 contest is still too incredible to be explained by luck or cherry-picking. He was hot.

Hot hands don’t happen every day but they do happen.

If you enjoyed this post, here are some more of statistician Gary Smith‘s reflections on the odd ways that numbers rule in sports:

The World Series: What the luck? Who will win the World Series? I don’t know, but I do know that baseball is the quintessential game of luck

The paradox of luck and skill: Why did Shane Lowry win the British Open golf championship? Because someone had to

Also: Bridge: Why shuffle the deck seven times? For years, competitive bridge players complained that computer shuffling of cards produced goofy results. Statisticians sided with the computers. Their reasoning might not be what you think.